"Happy Farm" PPT courseware 3 Simple campus recruitment activity planning plan summary enterprise and institution recruitment publicity lecture PPT template is a general PPT template for business post competition provided by the manuscript PPT, simple campus recruitment activity planning plan summary enterprise and institution recruitment promotion Lecture PPT template, you can edit and modify the text and pictures in the source file by downloading the source file. If you want more exquisite business PPT templates, you can come to grid resource. Doug resource PPT, massive PPT template slide material download, we only make high-quality PPT templates!
| 文件名 如何下载使用 | 下载次数 | Download Points | 下载地址 |
|---|---|---|---|
| "Happy Farm" PPT coursew... | 14925次 | 0.00 | Free Download |
Tips: If you open the template and feel that it is not suitable for all your needs, you can search for related content "Happy Farm" PPT courseware 3 is enough.
How to use the Windows system template
Directly decompress the file and use it with office or wps
How to use the Mac system template
Directly decompress the file and use it Office or wps can be used
Related reading
For more detailed PPT-related tutorials and font tutorials, you can view: Click to see
How to create a high-quality technological sense PPT? 4 ways to share the bottom of the box
Notice
Do not download in WeChat, Zhihu, QQ, built-in browsers, please use mobile browsers to download! If you are a mobile phone user, please download it on your computer!
1. The manuscript PPT is only for study and reference, please delete it 24 hours after downloading.
2. If the resource involves your legitimate rights and interests, delete it immediately.
3. Contact information: service@daogebangong.com
"Happy Farm" PPT courseware 3, due to usage restrictions, it is only for personal study and reference use. For commercial use, please go to the relevant official website for authorization.
(Personal non-commercial use refers to the use of this font to complete the display of personal works, including but not limited to the design of personal papers, resumes, etc.)

Related reading
For more detailed PPT-related tutorials and font tutorials, you can view:Please click to see







Authoritative PPT Summary
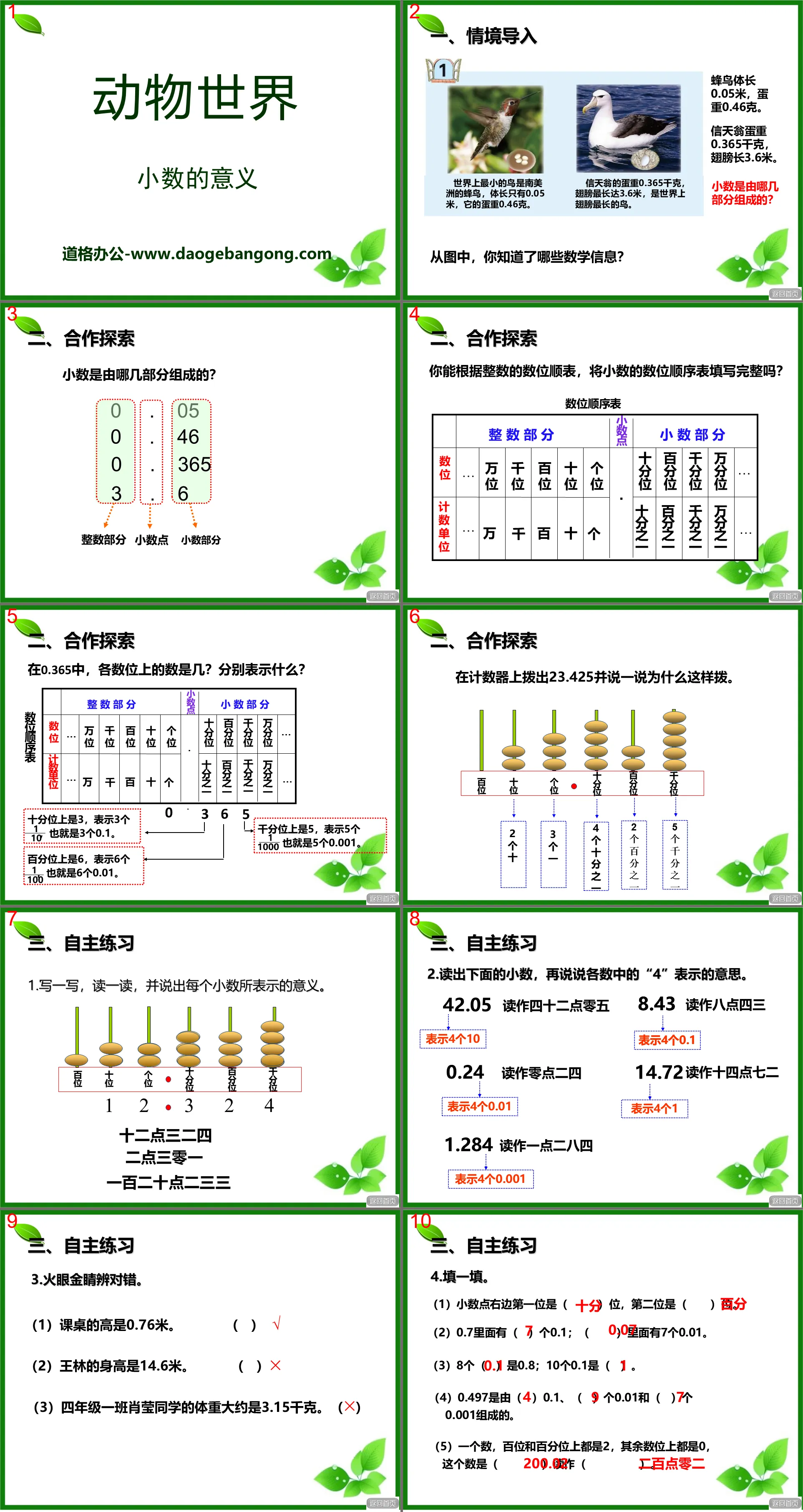
"Happy Farm" PPT courseware 3
80÷20=4
(80×2)÷(20×2)=4
(80×5)÷(20×5)=4
(80×10)÷(20×10)=4
Looking from top to bottom, both the dividend and the divisor expand by the same multiple.
It is found that the first set of calculations all yields 4
(80÷2)÷(20÷2)=4
(80÷4)÷(20÷4)=4
(80÷10)÷(20÷10)=4
Looking from top to bottom, both the dividend and the divisor are reduced by the same multiple.
It is found that the results of the second set of equations are all 4, and the quotient remains unchanged.
Can you try to sum up these two situations in one sentence?
The dividend and divisor expand or contract simultaneously by the same multiple, and the quotient remains unchanged.
Is this pattern discovered by the students universal? Could you please give a few more examples to see how the dividend and divisor expand or contract at the same time by the same multiple, and the quotient remains unchanged?
Just now, through observation, thinking, discussion, and verification, the students confirmed that in division, the dividend and divisor expand or contract by the same multiple at the same time, and the quotient remains unchanged. Can anyone name the pattern we discovered?
This law is usually called: "the law of constant commerce".
I look at the first question. Because the dividend and the divisor do not expand or contract at the same time, even though the multiples are the same, the quotient still changes.
In the second and third questions, although the dividend and the divisor expand or contract at the same time, the quotient changes because the multiples are different.
Question 4: The dividend and divisor do not expand at the same time, but increase by the same number at the same time, so the quotient also changes.
Now if you look at "The Law of Invariable Business", which words do you think are particularly important?
The dividend and divisor expand or contract simultaneously by the same multiple (except 0), and the quotient remains unchanged.
Classmates, whose smile is the smartest one and why?
First time: 6 3
Second time: 60 30
The third time: 600 300
The little monkey's smile is a smart smile, because more and more little monkeys are getting peaches.
The monkey king's smile is a wise smile. Because the monkey king used the constant law of business to deceive the little monkeys, each little monkey still got 2 peaches.
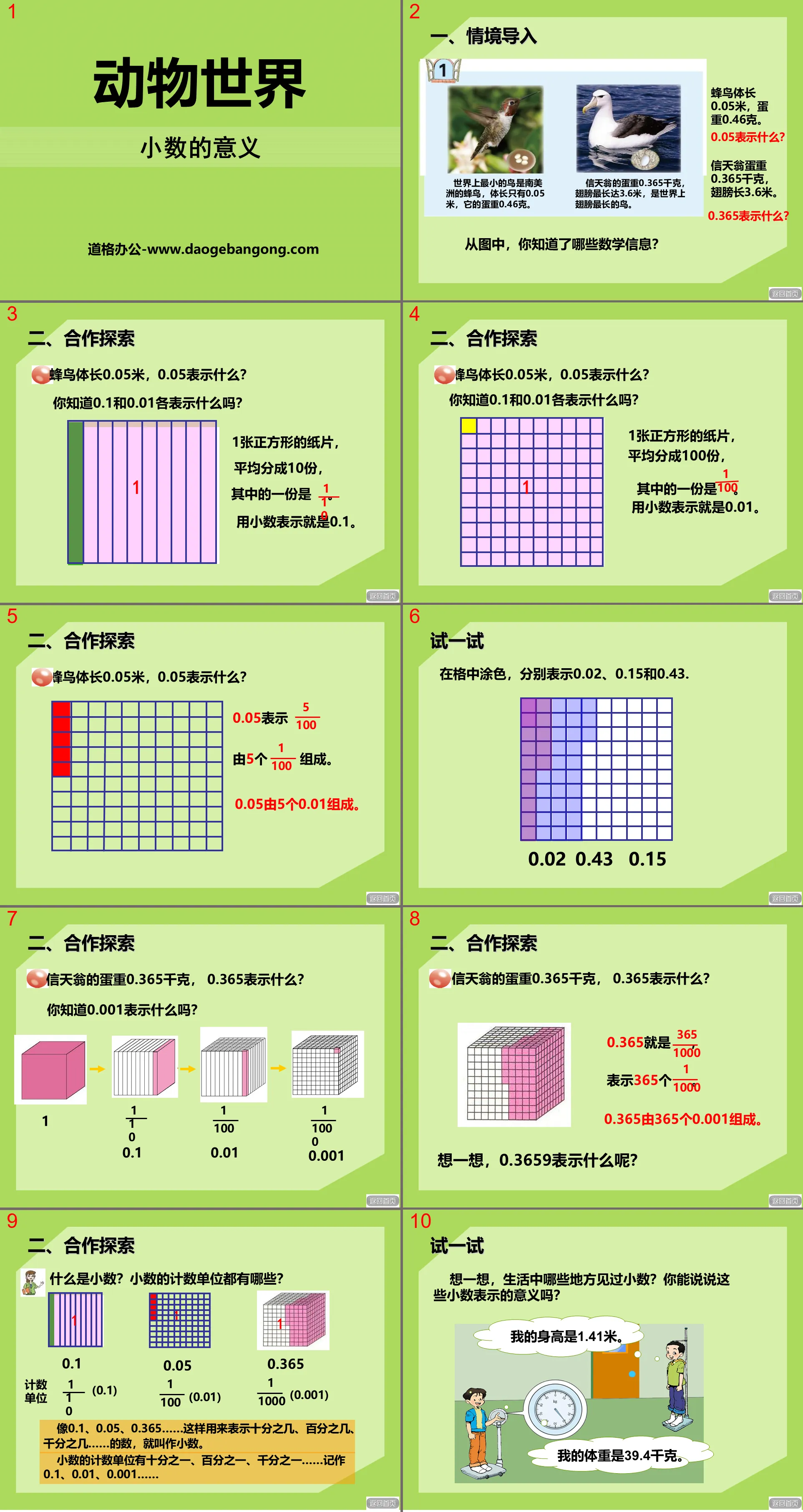
The following is the process of Naughty calculating "400÷25". Carefully observe every step of the calculation. What inspired you?
400÷25
= (400 × 4) ÷ (25 × 4)
=1600÷100
=16
Can you use this method to calculate the following questions?
150÷25
=(150 ×4)÷(25 ×4)
=600÷100
=6
Keywords: Happy Farm teaching courseware, Qingdao edition fourth grade mathematics volume PPT courseware download, fourth grade mathematics slide courseware download, Happy Farm PPT courseware download, .PPT format;
For more information about the "Happy Farm" PPT courseware, please click on the "Happy Farm ppt" tab.
"Happy Farm" PPT courseware 7:
"Happy Farm" PPT courseware 7
1. Situation introduction
There are 56 hollies, 72 willows, and 28 poplars.
How many saplings were purchased in total?
There are 80 roses, 88 peonies, and 112 camellias.
How many flower seedlings did you purchase in total?
Based on this information, you can...
"Happy Farm" PPT courseware 6:
"Happy Farm" PPT courseware 6
1. Situation introduction
Purchased 20 bags of flower soil, 25 bags per bag, 2 kilograms per bag.
How many kilograms of flower soil were purchased in total?
Purchased 10 bags of flower fertilizer, 8 bags per bag, 5 kilograms per bag.
How many kilograms of flower fertilizer were purchased in total? ..
"Happy Farm" PPT courseware 5:
"Happy Farm" PPT courseware 5
Class goals:
1. In the process of solving problems, discover and understand the distributive law of multiplication through mathematical activities such as observation, conjecture, verification, induction, and reasoning. (emphasis)
2. Be able to flexibly use the distributive law of multiplication to simplify...