"Determining a quadratic function from the coordinates of three non-collinear points" PPT

Description

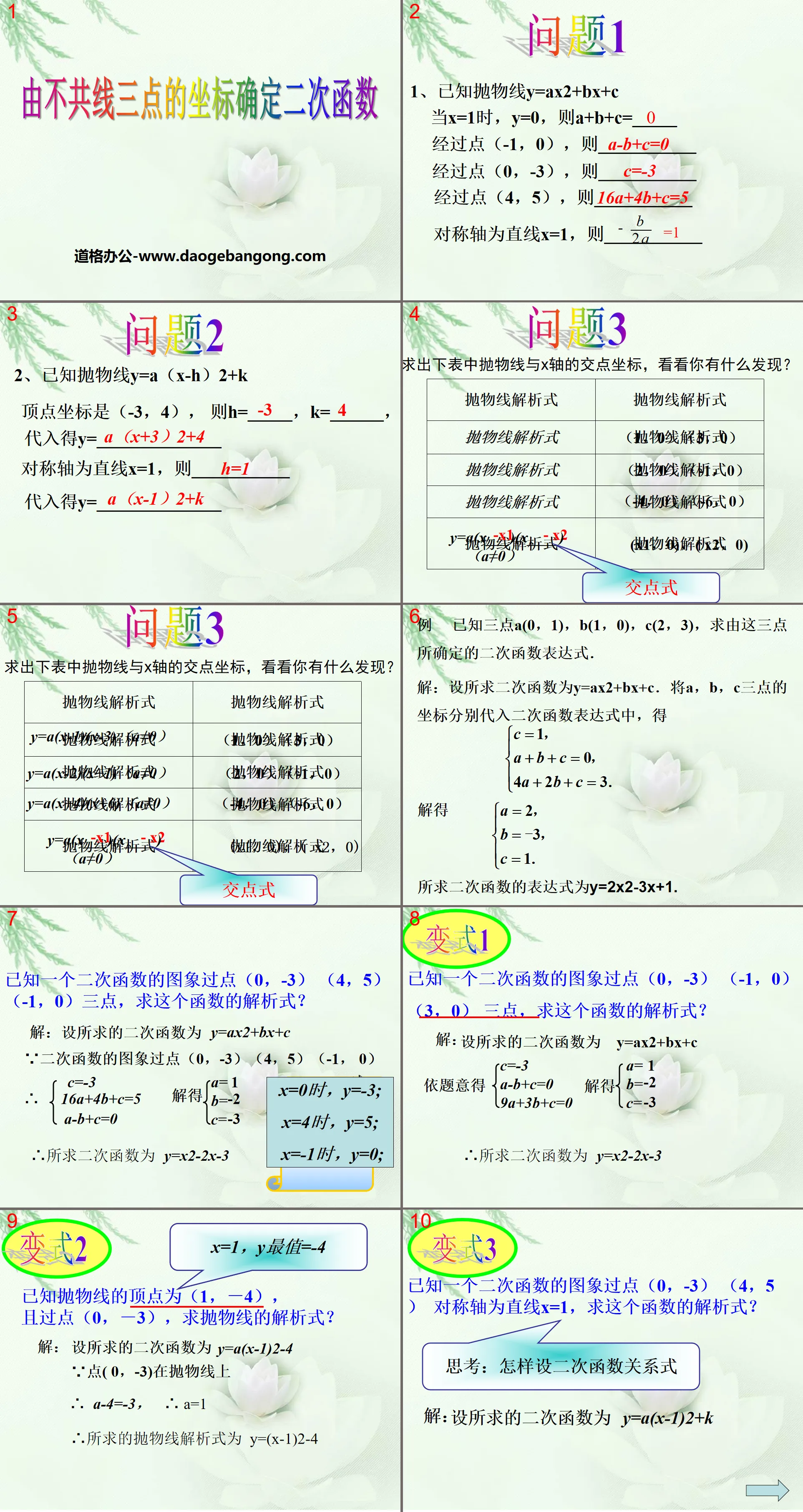
"Determining a quadratic function from the coordinates of three non-collinear points" PPT

Part One: Problem Exploration

Question 1

1. It is known that the parabola y=ax2+bx+c

When x=1, y=0, then a+b+c=_____

After passing the point (-1, 0), then ___________

After passing the point (0, -3), then ___________

After passing the point (4, 5), then ___________

The axis of symmetry is the straight line x=1, then ___________

2. It is known that parabola y=a(x-h)2+k

The vertex coordinates are (-3, 4), then h=_____, k=______,

Substituting in, we get y=______________

The axis of symmetry is the straight line x=1, then ___________

Substituting in, we get y=______________

Determine the quadratic function PPT from the coordinates of three non-collinear points, part 2: analysis of examples

Example Given three points A(0,1), B(1,0), C(2,3), find the expression of the quadratic function determined by these three points.

Solution: Suppose the quadratic function sought is y=ax2+bx+c. Substituting the coordinates of the three points A, B, and C into the quadratic function expression, we get

The expression of the quadratic function sought is y=2x2-3x+1.

It is known that the graph of a quadratic function passes through the point (0,-3) (4,5)

(-1, 0) three points, find the analytical formula of this function?

Solution: Suppose the quadratic function required is y=ax2+bx+c

∵The graph of the quadratic function passes through the points (0, -3) (4, 5) (-1, 0)

∴c=-3

16a+4b+c=5

a-b+c=0

Solutions have to

a=1

b=-2

c=-3

∴The quadratic function required is y=x2-2x-3

Determine the quadratic function PPT from the coordinates of three non-collinear points, the third part: several commonly used analytical formulas for quadratic functions

General formula y=ax2+bx+c (a≠0)

Given three pairs of corresponding values ​​of three point coordinates, select the general formula

Vertex formula y=a(x-h)2+k (a≠0)

Known the vertex coordinates or axis of symmetry or maximum value, select the vertex formula

Intersection formula y=a(x-x1)(x-x2) (a≠0)

Given the coordinates of the two intersection points of the parabola and the x-axis, select the intersection formula

When using the undetermined coefficient method to determine the analytical expression of a quadratic function, a function expression should be appropriately selected based on the characteristics of the conditions.

Keywords: Free download of Hebei Education Edition mathematics PPT courseware for the second volume of the ninth grade, quadratic function PPT download determined by the coordinates of three non-collinear points, .PPT format;

For more information about the PPT courseware "Determining a quadratic function from the coordinates of three non-collinear points", please click the Determination of a quadratic function from the coordinates of three non-collinear points ppt tag.

File Info

Update Time: 2024-11-14

This template belongs to Mathematics courseware Hebei Education Edition Ninth Grade Mathematics Volume 2 industry PPT template

Preview